Ancient Egyptians calculated by unit fractions, such as 1/2, 1/3, 1/4, 1/10, .... The hieroglyph for an open mouth was used to DENOTE A FRACTION, with a number hieroglyph written below this "open mouth" icon to DENOTE DENOMINATOR OF THE FRACTION. Any FRACTION we write with a non-UNIT NUMERATOR was written by ancient Egyptians as a SUM OF UNIT FRACTIONS.
These UNIT FRACTIONS have, therefore, become known as "Egyptian Fractions". We use them every day in making change for a dollar: 1 penny => $1/100; 1 nickel => $1/20; 1 dime => $1/10; 1 quarter => $1/4; 1 half-dollar => $1/2.
The great British mathematician, J. J. Sylvester (1814-197) -- whom we identified in another file as the tutor of the young Florence Nightingale -- developed AN ALGORITHM FOR CONVERTING ANY NON-UNIT FRACTION TO THE SUM OF UNIT OR EQYPTIAN FRACTIONS.
- Given a fraction, such as 7/24, find the LARGEST EGYPTIAN FRACTION JUST LESS than 7/24.
- Find this by performing DIVISION: 24
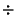7 = 3 + REMAINDER 3. So 1/4 = 6/24 is the ANSWER. - Perform SUBTRACTION: 7/24 - 1/4 = 7/24 - 6/24 = 1/24.
- Hence, 7/24 = 1/4 + 1/24.
Again,
- Given 2/35, find the LARGEST EGYPTIAN FRACTION JUST LESS than 2/35.
- DIVISION: 35 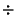2 = 17 + REMAINDER 1.
So 1/18 is the ANSWER.
- 2/35 - 1/18 = (2 · 18)/(35 · 18) - (1 · 35)/(18 · 35) = 36/630 - 35/360
= 1/360.
- Hence, 2/35 = 1/18 + 1/630.
Etc.
- Given 2/35, find the LARGEST EGYPTIAN FRACTION JUST LESS than 2/35.